This post digs into Lecture 5 of Paul Meehl’s course “Philosophical Psychology.” You can watch the video here. Here’s the full table of contents of my blogging through the class.
It’s impossible for me to fathom, but the molecular theory of matter wasn’t widely accepted until the 1910s. Very famous, very smart scientists, including Ernst Mach and Henri Poincare, thought atoms were merely a convenient fiction for predicting experimental outcomes. Statistical calculations, like those deployed to derive the ideal gas laws from kinetic theory, were akin to approximating integrals with discrete sums. Since bulk media obeyed differential equations, many thought it was more likely that they were continuous substances. That nature was an assemblage of a nearly infinite collection of invisible, discrete billiard balls seemed rightfully outlandish.
The controversy was effectively put to rest by Jean Baptiste Perrin in his 1913 book Les Atomes. Perrin spent hundreds of pages detailing the experimental evidence for atoms and molecules. The core of his argument was that if you assume molecules exist, you could count them in surprisingly diverse ways.
Again, it’s difficult to take my 21st century brain and imagine the mindset of an eighteenth century chemist, but scientists had somehow accepted the unit of a mole before they accepted the theory of atoms. The person who coined the term mole, Nobel Prize Winner Wilhelm Ostwald, was another famous atomic skeptic. How could you believe in moles but not molecules? I suppose it’s not that crazy. Different substances had different “molecular weights,” meaning that the same “amount of stuff” could weigh different amounts. You could observe nitrogen and hydrogen balloons at the same temperature, pressure, and volume; one would rise while the other would not. Oil floats on water. Gold is heavier than lead.
Perrin argued a mole always had the same number of molecules. This number, NA, is called Avogadro’s Constant and equals 6.02214076 x 1023.1 No matter how he went about trying to estimate NA, he always found the same answer.
Perrin presented a derivation of NA from Brownian motion, assuming a macroscopic particle was bombarded by microscopic particles in a fluid. This bombardment caused the macroscopic particle to randomly move around. The predictions of Brownian motion would rely on some number of molecules in any given unit of the fluid. Balancing the predictions of Brownian motion against the effect of gravity gave the number of water molecules in a small volume. Extrapolating out provided an estimate of NA.
Perrin described a derivation of Einstein that predicted the color of the sky by analyzing the statistical mechanical scattering of light by air particles. This derivation relied on an estimate of the number of particles in a given volume of air. Perrin derived an estimate from the theory of black body radiation, using their calculation of Boltzman’s constant and applying the fact that the “R” in the ideal gas law was equal to Avogadro’s number divided by Boltzman’s constant.
Perrin also used properties of alpha decay to compute an estimate. Radium emits alpha particles, which combine with electrons to produce helium. Perrin knew the rate of alpha particle decay, which yielded a prediction of the number of Helium molecules. He then compared this number to the amount of Helium produced to get yet another estimate of NA.
Electrochemistry determined the charge required to deposit one mole of silver onto an electrode through electrolysis. This was called a Faraday and had value F. Assuming it costs one electron to deposit one atom, then the total number of atoms deposited should be F/e where e is the charge of the electron. Millikan had recently computed an estimate for e, and hence this gave another path to estimating Avogadro’s number.
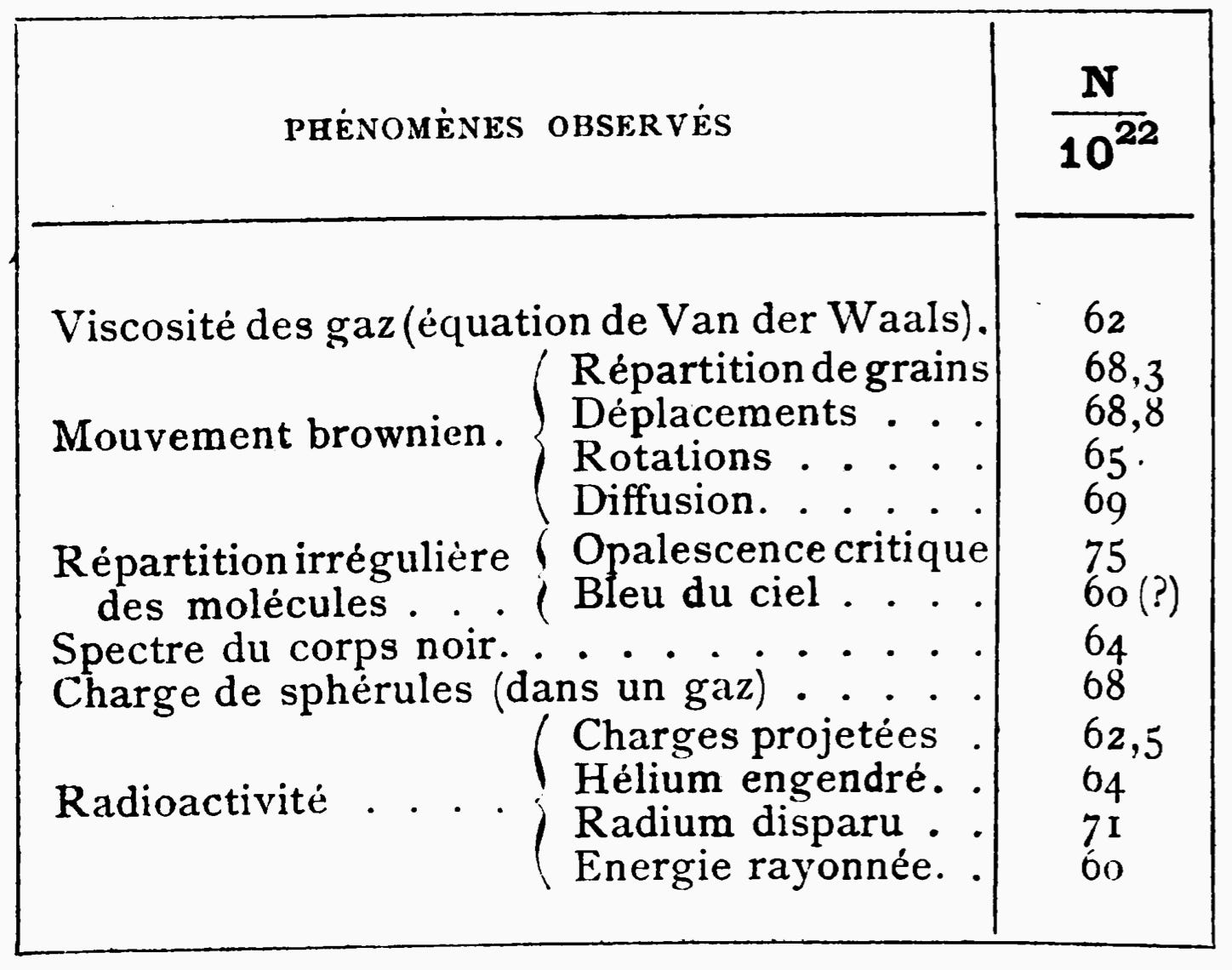
The conclusion of Les Atomes contains a remarkable table. Perrin lists his estimates of NA from 13 different derivations.
No matter which he chose, the number was always around 6x1023. The fact that all of these different calculations gave the same answer was indeed a damned strange coincidence. After Perrin’s work, almost everyone (except Mach2) conceded that matter was composed of atoms and molecules. This was in 1913! Look where we are today.
Let’s take a minute to explore how this example fits into Meehl’s Lakatosian Defense framework and why Meehl spent so much time on Avogadro in Lecture 5. Here we have a single theory: “A mole is a constant number of molecules.” Adjoining this theory to a variety of different derivation chains gives the same value for the NA. The results are too close to each other for that to have happened by pure chance, and hence the theory is corroborated.
Well, except it’s not really that clean. First, it’s hard to precisely say what is the probability here. What does it mean that the probability that Perrin’s calculations all gave the same NA was exceptionally small if atoms weren’t real? What is p(atoms)? The atomic skeptics were clearly very surprised by Perrin’s results. Wesley Salmon quotes Poincare as exclaiming, “How can you argue, if all of the counts come out the same?” He announced, “Atoms are no longer a useful fiction; things seem to us in favor of saying that we see them since we know how to count them.”
Still, I don’t think we can make this “probability” notion too formal. Meehl chirps against hypothesis testing here. It’s certainly not the case that physicists ran some F-test or something on Perrin’s table to convince themselves of the result. It certainly wasn’t the case that scientists at the time ground out some Bayesian confirmation calculation, as Bayesian statistics wouldn’t be formulated for another twenty years. Salmon tries to construct a post hoc “common cause” formalization of atomic confirmation, but I am not at all convinced by his handwavy argument.
Meehl argues it’s the specificity of the predictions that convinced everyone. I suppose we could reverse engineer a Meehlian Spielraum argument here. Suppose Perrin had instead assumed that Avogadro’s number was 6x1023. Then he’d get estimates of the charge of the electron, Boltzmann’s constant, the decay rate of radium, and a dozen other physical constants, all within a factor of 25% of their known value. Perrin’s atomic theory would be predicitng a remarkably narrow range of the Spielraum over dozens of experiments. Again, this isn’t what Perrin did, but I can imagine that if he had presented his results this way, people would have been just as convinced.
And obviously, the fact that all of Perrin’s rested on a single number made the whole theory too good to ignore. A friend joked this weekend that these days, we’re happy if a billion-parameter model gets one prediction correct. But we’re much certainly happier if one parameter yields a billion predictions.
Indeed, the atomic theory would continue to predict the outcomes of endless experiments. It would prove critical in understanding X-rays and estimating their wavelengths. And once the atomic theory became entrenched, it would be guarded by Lakatosian Defense. When Bäcklin and Bearden made measurements that found too high a value for X-ray wavelengths, this suggested an error in the estimates of Avogadro's number. Prins would write to Nature to correct the record: “the usual diffraction formula needs a correction when applied to X-rays under the usual experimental conditions.” Atomic theory was now far too useful and had to be defended at all costs.
If you want to get more into the weeds of atoms, check out Wesley Salmon’s Scientific Explanation and the Causal Structure of the World. The blog here pieces together Meehl’s lecture, Salmon’s argument, and my own reading of Perrin. Maybe I got too into the weeds here.
In our modern standards, Avogadro’s constant defines a mole. It is now completely backwards: A mole was first defined by a volume of gas, but now defined to be NA molecules.
As Meehl says, Mach died in 1916, but maybe would have changed his mind had he lived a bit longer.


Interesting analogy here with physicists regarding heliocentrism as a convenient fiction for more accurate prediction calculations than geocentrism, for decades after Copernicus, since heliocentrism obviously made no physical sense and violated everything we knew about everything.
(This is covered at length in Kuhn's _Copernican Revolution_, which is in many ways a better book than _Scientific Revolutions_, although also less significant.)
An interesting take from a "Philosophical Psychology" viewpoint. Practising empiricists had long been dismissed for shallow "Phenomenologists" by Physicists but eventually prevailed on the sheer weight of accumulated evidence over which Perrin agonises in the 20th Century almost 200 years later.